Maths - Pentagon

I was looking for info on the properties of pentagons for a geometry question in my textbook.

In textbook, we are given a pentagram (5 sided star) drawn inside a regular pentagon (equal sides) and have to work out the angles.

Image source:  maths.surrey.ac.uk

Unfort. the info for this problem is neither within the chapter, nor the book and the answers are not provided,  so I had a look on the internet and found that each of the 5 interior angles of the pentagon are 108 degrees .  The interior angles all add up to 540 degrees.  

I worked out by deduction that as all sides are = therefore all angles will be =.  In the way the sides of the pentagram would be equal and the outer layer of angles would be =.  As each vertex of the pentagram divides each corner angle on the pentagon into 3 - each outer angle of the pentragram would be 180 /3 = 36 degrees.

Therefore all of the angles along the pentagon are 36 degrees.

I double checked this with my protractor (which was not part of the question) and they are 36  degrees.  I also checked the other angles and they are either 72 degrees of 108 degrees.

This diagram has information on it that confirms this deduction i.e  Other angles within the pentagram would be multiples of 36 i.e. either 72 degrees or 108 degrees depending:

Source:  http://eyephi.com/oneeye/what-followed-after-my-discovery/

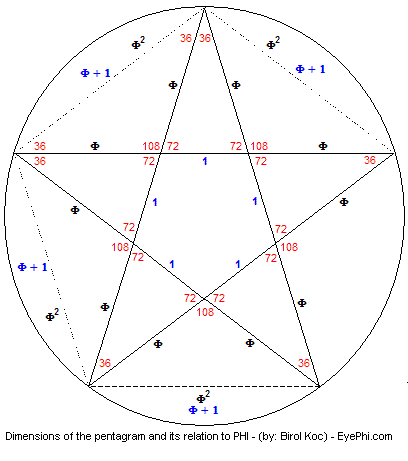
This is another image I found:

Image Source:  http://www.aamt.edu.au/digital-resources/R11195/transcript.html 

What was interesting is this image I found where the concept is repeated again in the centre and again.

Image source:  aleph0.clarku.edu

This is all based on all the sides being equal and so all the angles being equal.

I found the info below on http://www.mathopenref.com/pentagon.html

Properties of regular pentagons

Interior angle	108°	Like any regular polygon, to find the interior angle we use the formula   (180n–360)/n . For a pentagon, n=5. See Interior Angles of a Polygon
Exterior Angle	72°	To find the exterior angle of a regular pentagon, we use the fact that the exterior angle forms a linear pair with the interior angle, so in general it is given by the formula 180-interior angle. SeeExterior Angles of a Polygon
Area	1.72 S2 approx	Where S is the length of a side. To find the exact area of a regular pentagon or any regular polygon, using various methods, see Area of a Regular Polygon and Area of an Irregular Polygon

Properties of all pentagons

Number of diagonals	5	The number of distinct diagonals possible from all vertices. (In general  ½n(n–3)  ). In the figure above, click on "show diagonals" to see them. See Diagonals of a Polygon
Number of triangles	3	The number of triangles created by drawing the diagonals from a given vertex. (In general n–2). In the figure above, click on "show triangles" to see them. See Triangles of a Polygon
Sum of interior angles	540°	In general 180(n–2) degrees . See Interior Angles of a Polygon

There is also some info on pentagons/pentgrams and crop circles here:  http://www.axve02.dsl.pipex.com/cropcirc/chap5/Pentagram.htm

Even more about pentagrams:  http://mathworld.wolfram.com/Pentagram.html

http://www.anselm.edu/homepage/dbanach/31-drawing.htm (This includes various drawings as well as pentagrams)

Other info on pentagrams:

The pentagram, is also called the 5-point star, pentacle, pentalpha, or pentangle, is the star polygon .(See diagram below).

It is a pagan religious symbol that is one of the oldest symbols on Earth and is known to have been used as early as 4000 years B.C. 

The first known uses of the pentagram (the simplest regular star polygon) are found in Mesopotamian writings dating to about 3000 BC. The 5 points represented the 5 astrological directions associated with Jupiter, Mercury, Mars and Saturn, and Venus. 

It is a symbol with its roots in alchemy and in the ancient mysteries.  The metaphorical interpretation of geometric figures goes back to Pythagoras and the beginnings of philosophy.
In alchemical symbolism the five points signify the interconnection of the four elements (Air, Fire, Water, and Earth) united by the fifth element or "quintessence" that is sometimes called Aether and more often Spirit. 

The pentagram is used in traditional Chinese medicine and Taoism too, which employs a system of five elements rather than four (Water, Wood, Metal, Fire, Earth).  The fact that we have five digits on each of our hands and feet is another way that five becomes a mysterious number signifying humanity.  More recently, the pentagram has been widely publicized as the chief symbol of Wicca

Other star polygons:  

Image Source:  http://mathworld.wolfram.com/Pentagram.html

Other info on the pentagons, pentagram and organisations:
USA Pentagon Building
Free masons - Pentagram (5 senses)
See this page:  http://www.lakeharrietlodge.org/lhl277/MainMenu/Home/MasonicEducation/TheSymbolismofthePentagram/tabid/413/Default.aspx


Sacred Geometry:  http://www.esotericonline.net/group/sacredgeometry/forum/topics/sacred-geometrythe-pentagram?commentId=3204576%3AComment%3A9364&groupId=3204576%3AGroup%3A9042